Mathematics: Fun tricks for everybody

Mathematics: Fun tricks for everybody, available at Free, has an average rating of 4.85, with 15 lectures, based on 107 reviews, and has 10393 subscribers.

You will learn about Creative problem solving, with fun tricks and geometrical illustrations of the results. How to derive the formula for the sum of squares of all natural numbers from 1 to n. How to derive the formulas for partial sums for two very important examples of arithmetic progressions: 1+2+…+n and 1+3+5+…+(2n-1). How to derive the general formula for partial sums of geometric progressions; an important example of the sum of all the natural powers (from 1 to n) of 1/2. How to derive some less known formulas, using cool computational tricks. How to prove divisibility in some simple cases, using very basic (but fun) reasoning. You will learn (from an article) how to convert infinite periodic decimal expansions into fractions, by smart substitutions and solving linear equations. You will learn (from an article) some basics about continued fractions (CF), and how to recognise irrational surds from their infinite periodic CF-expansions. You will learn (from an article) about an interesting family of nested square roots and how to prove that some members of this family are… integers! This course is ideal for individuals who are High-school students who want to see some fun maths stuff they won't see at school. or Everybody who wants to learn some fun maths tricks and see some nice geometrical illustration of mathematical formulas. It is particularly useful for High-school students who want to see some fun maths stuff they won't see at school. or Everybody who wants to learn some fun maths tricks and see some nice geometrical illustration of mathematical formulas.

Enroll now: Mathematics: Fun tricks for everybody

Summary

Title: Mathematics: Fun tricks for everybody

Price: Free

Average Rating: 4.85

Number of Lectures: 15

Number of Published Lectures: 15

Number of Curriculum Items: 15

Number of Published Curriculum Objects: 15

Original Price: Free

Quality Status: approved

Status: Live

What You Will Learn

Creative problem solving, with fun tricks and geometrical illustrations of the results.
How to derive the formula for the sum of squares of all natural numbers from 1 to n.
How to derive the formulas for partial sums for two very important examples of arithmetic progressions: 1+2+…+n and 1+3+5+…+(2n-1).
How to derive the general formula for partial sums of geometric progressions; an important example of the sum of all the natural powers (from 1 to n) of 1/2.
How to derive some less known formulas, using cool computational tricks.
How to prove divisibility in some simple cases, using very basic (but fun) reasoning.
You will learn (from an article) how to convert infinite periodic decimal expansions into fractions, by smart substitutions and solving linear equations.
You will learn (from an article) some basics about continued fractions (CF), and how to recognise irrational surds from their infinite periodic CF-expansions.
You will learn (from an article) about an interesting family of nested square roots and how to prove that some members of this family are… integers!

Who Should Attend

High-school students who want to see some fun maths stuff they won't see at school.
Everybody who wants to learn some fun maths tricks and see some nice geometrical illustration of mathematical formulas.

Target Audiences

High-school students who want to see some fun maths stuff they won't see at school.
Everybody who wants to learn some fun maths tricks and see some nice geometrical illustration of mathematical formulas.

Mathematics is often thought of as a tool for engineering or finances, but it is not its only purpose. Mathematics is also a product of the human mind which has an aesthetic function: to satisfy the human need of completeness, to give intellectual satisfaction, and even delight.

This course shows mathematics as art, not as craft. It can help you fall in love (possibly: again) with mathematics, or at least help you understand the math lovers who always praise the beauty of maths.

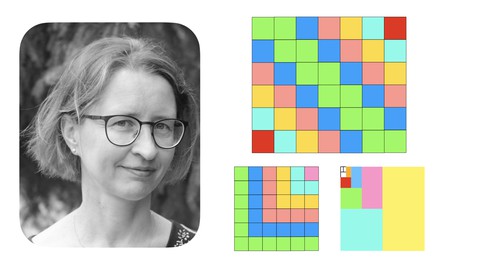
All the problems solved in this course are solved with help of really simple tricks, without using complicated theories. Some of them were presented in my earlier (free) course “Mathematics: Proofs by induction”. In this earlier course, the formulas were proven by induction, while in the present course they are *derived*, so that you don’t need to know the result in advance, which you really needed in case of proofs by induction. In some cases you will also get geometrical interpretations of the obtained results (yes: the three rectangles in the course image).

Because of the time restriction (max 2 hours of video content) for free courses, some problems are solved in articles added as resources for the course.

All the problems are on high-school level, but the course can be interesting for anybody who wants to see some recreational mathematics and learn some neat tricks.

The following types of problems are presented here:

In Section 1:

Several formulas for sums of elements in sequences of numbers: some arithmetic progressions (sum of all the natural numbers from 1 to n in Lecture 2, sum of all the odd numbers from 1 to 2n-1 in Lecture 3), a geometric progression (Lecture 8), the sequence of the squares of natural numbers (Lecture 4), two less known sequences (in Lectures 5 and 6); in three cases (Lectures 2, 3, and 8) you get nice geometrical interpretations of the results. One formula for a product of elements in a sequence of numbers (in Lecture 7).

In Section 2:

Two divisibility problems (Lectures 9 and 10) and one inequality (Lecture 11).

In Section 3:

In the last section (articles only, no videos) you will get introduced to three different situations where a similar trick is applied: getting rid of infinitely many operations by applying some smart substitutions, and solving some equations (linear or quadratic, with one unknown):

— infinite periodic decimal expansions (Lecture 12)

— infinite periodic continued-fraction expansions (Lecture 13)

— infinitely many times nested square roots (Lecture 14).

Extras

You will learn: about all the courses we offer. You will also get a glimpse into our plans for future courses, with approximate (very hypothetical!) release dates.

Course Curriculum

Chapter 1: Derivations of some formulas

Lecture 1: Introduction to the course

Lecture 2: Derivation of a formula, Problem 1

Lecture 3: Derivation of a formula, Problem 2

Lecture 4: Derivation of a formula, Problem 3

Lecture 5: Derivation of a formula, Problem 4

Lecture 6: Derivation of a formula, Problem 5

Lecture 7: Derivation of a formula, Problem 6

Lecture 8: Derivation of a formula, Problem 7

Chapter 2: Some proofs without induction

Lecture 1: Proving divisibility without using induction, Problem 1

Lecture 2: Proving divisibility without using induction, Problem 2

Lecture 3: Proving inequalities without using induction, Problem 3

Chapter 3: Three groups of problems where we avoid infinite repetitions in a smart way

Lecture 1: Infinite periodic decimal expansions, Problem 1

Lecture 2: Infinitely repeating continued fractions, Problem 2

Lecture 3: Infinitely repeating square roots, Problem 3

Chapter 4: Extras

Lecture 1: Bonus Lecture

Instructors

Hania Uscka-Wehlou
University teacher in mathematics, PhD
Martin Wehlou
Editor at MITM AB

Rating Distribution

1 stars: 0 votes
2 stars: 3 votes
3 stars: 3 votes
4 stars: 17 votes
5 stars: 84 votes

Frequently Asked Questions

How long do I have access to the course materials?

You can view and review the lecture materials indefinitely, like an on-demand channel.

Can I take my courses with me wherever I go?

Definitely! If you have an internet connection, courses on Udemy are available on any device at any time. If you don’t have an internet connection, some instructors also let their students download course lectures. That’s up to the instructor though, so make sure you get on their good side!

Menu